5.1.3. Seeing and telescope aperture

Since the atmospherie-induced wavefront error - so called seeing error - changes with (D/r0)5/6, it will vary, for given atmospheric coherence length (Fried parameter) r0, with the aperture size D.

Eq. 52.1 and 52.2 give following averaged RMS errors in the average 2 arc seconds seeing (r0~70mm for 550nm), over the span of amateurs' apertures, with the corresponding Strehl values calculated from the seeing Strehl approximations:
 

ERROR/APERTURE (mm)		50	100	150	200	300	400	500
D/r0		0.71	1.43	2.14	2.86	4.29	5.71	7.14
RMS	Short exposure (roughness)	0.044	0.078	0.109	0.139	0.194	0.246	0.3
	Long exposure (tilt + roughness)	0.12	0.22	0.3	0.39	0.54	0.69	0.84
Strehl	Short exposure	0.93	0.79	0.64	0.48	0.24	0.11	0.043
	Long exposure	0.61	0.28	0.16	0.11	0.05	0.03	0.02

While these figures appear rather pessimistic, one should keep in mind that they are only averaged values. Statistically, one of many possible break-down's for the dismal 0.69 wave RMS long-exposure error at (D/r0)=5.7 (for 400mm aperture in 2" seeing) is 1.38 and 0.34 wave, half the time each. Likewise, its short exposure error splits to 0.5 and 0.12 wave RMS. Placing the visual error level roughly in between the two would give 0.94 and 0.23 wave RMS. This means that half the time the image would be a real mess, but the other half it would be significantly better, with the visual Strehl of around 0.35, comparable to little over 1/2 wave P-V of spherical aberration.

Also, the tilt error effect is not detrimental at very small apertures (smaller than ~4") where, in average seeing, it causes image to move about randomly as a whole, relatively slowly, without significantly  affecting its visual quality. Thus, in this aperture range, the size of seeing error in visual observing is mainly determined by the wavefront roughness component (Eq. 52.2).

And, since these figures are based on the standard Kolmogorov model, which assumes an infinite outer scale of turbulence, the actual error is somewhat smaller for the long-exposure modality due to the smaller tip-tilt error component with a finite actual scale of turbulence.

As the aperture increases - or the seeing worsens - the eye has more difficulty to keep up with the frequency of image motion, as the diffraction pattern gradually breaks into speckle structure, with an increasing number of  pattern components moving randomly within the speckle envelope. The result is progressive blurring and bloating of the star image. The roughness error component too increases with (D/r0)5/6 until, at a sufficiently large aperture - or in sufficiently bad seeing - diffraction pattern disintegrates into a random speckle structure (FIG. 77). The illustrations show pattern structure as a short-exposure snapshot; physical size of the Airy discs is identical (i.e. focal ratio is the same for all apertures) to make the atmospheric effect directly scalable.

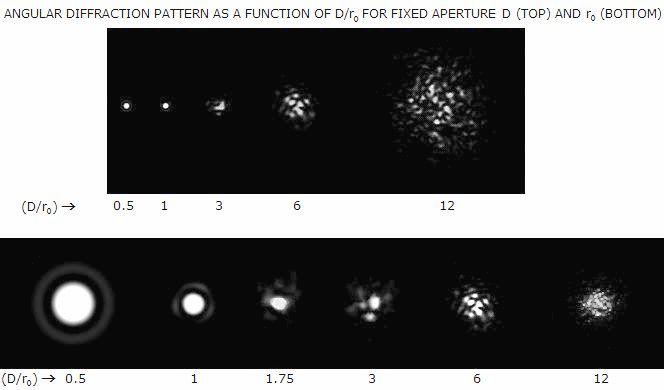
The speckles, being formed by the energy coming from the fragments of the broken wavefront over the pupil that meet in phase at the points randomly scattered around Gaussian image point, approximate in size diffraction disc (this apply to the brightest, so called first-order speckles; fainter speckles are smaller in size). The diameter of the 1st-order speckles envelope, the bright central core of the entire star image, roughly analogous to the FWHM (Full-Width-at-Half-Maximum) of the PSF of aberration-free diffraction image of an aperture of diameter equal to r0, is approximated by λ/r0 in radians. This means that it is r0, not the aperture, that sets the resolution limit. The speckle phenomenon is not only characteristic of large professional telescopes. Even medium-size amateur telescopes in bad seeing begin to develop speckle structure.

Probably the simplest model to use for explaining the mechanism of speckle formation is to assume that the aperture of a telescope is, as a result of atmospheric turbulence, effectively broken into sub-apertures, each approximately of the diameter equal to r0, with a different, varying amounts of tilt. The resulting diffraction pattern is a product of superimposed sub-aperture patterns. Similarly to a multi-aperture telescope, the combined pattern shows tendency to segmentation - mostly in the rings area - with the number of first-order radial segments (spikes, lobes, etc.) approximately equaling that of sub-apertures. Unlike multiple-aperture telescope, the seeing-formed sub-apertures are in an optical disarray, poorly defined and in a constant motion. Thus the increase in number of "sub-apertures" quickly causes disintegration of the bright central disc and formation of the random speckle structure which is stationary only within time intervals smaller than ~20 milliseconds (short-exposure image).

Since speckles originate from wavefront roughness, which is proportional to (D/r0)5/6, their number will be larger for larger (D/r0) values, while their average angular size will be smaller in inverse proportion to the aperture size (thus, fully developed speckle pattern remains of similar angular size, regardless of aperture). The number of 1st-order speckles N can be approximated as a ratio of the area of the speckle envelope's FWHM (approximated, angularly, by λ/r0) vs. area of a single speckle (taking 2λ/D for the average angular separation of speckle centroids), or N~[(λ/r0)/(2λ/D)]2=(D/2r0)2; hence the spackle structure begins to form, at least statistically, at (D/r0)~3, and should become apparent at (D/r0)~4 and larger.

Note this approximation may somewhat overestimate the number of speckles, since based on r0, which is the seeing FWHM that includes the tilt error, i.e. random motion of the speckle structure around its central point.

Formation of the dynamic speckle structure changes the realm of a telescope; it voids its resolution limit, making it dependant on the atmosphere, not aperture size. Energy spread caused by it also significantly lowers telescope's contrast level. Since the error increases with aperture, given large enough difference in aperture size, there comes the level of seeing error which may take away the resolution/contrast advantage from the larger aperture and award it to the smaller one (FIG. 78).

This general scenario would play out with, say, 150mm, 300mm and 750mm apertures in 1, 2 and 4 arc seconds seeing (i.e. r0 of 144, 72 and 36mm, respectively).

Image below illustrates how angular size of diffraction pattern and its appearance change with the decrease in atmospheric coherence length r0 (top) and increase in aperture D (bottom).

Decrease in r0 for given aperture results in the progressive break up and enlargement of the diffraction pattern. For given r0, angular pattern size for (D/r0)>3 will be nearly constant, approximated by λ/r0. As D/r0 decreases below 3, the central maxima gradually regains its integrity, and limiting resolution shifts toward λ/D. Despite the central maxima becoming larger than speckles in larger apertures, its diameter becomes smaller than that of their primary speckle structure. Consequently, for 1.5<(D/r0)<2.5, the smaller aperture has better resolution than two or more times larger apertures. At (D/r0)~1, resolution level in the smaller and much larger (five or more times) apertures are similar; further decrease in aperture results in decreased resolution in smaller vs. larger apertures.

If we take for the smallest aperture D=50mm, and r0~100mm (~1.5 arc second seeing), then the bottom patterns, from left to right, correspond to 50, 100, 175, 300, 600mm and 1.2m apertures. Keep in mind that these represent averaged, statistical errors. In actual field conditions, as seeing fluctuates, 175mm aperture will have its D/r0 in the 1.5-2.5 range during periods of better seeing, out-resolving both smaller and larger apertures. Even 600mm aperture is likely to have moments when D/r0 drops to ~2.5, or somewhat bellow; that is, however, very unlikely for 1.2m aperture, which remains inferior to 150-300mm apertures (approximately) resolution-wise.

EFFECT ON CONTRAST

The degree of pattern integrity directly determines the maximum usable point-source magnification, defined as one needed to achieve limiting visual resolution allowed by seeing. Limiting magnification for extended object is more complex, varying with detail's inherent contrast, brightness and shape. In general, contrast of extended objects is affected more than point-source resolution - and particularly those with low inherent contrast - thus seeing-limited usable magnification for this type of objects should be somewhat lower. An indication of the effect of seeing on contrast and resolution in smaller vs. larger aperture is best presented with MTF graph (FIG. 80).

     This crude approximation using MTF model indicates that the smaller aperture would probably have relatively small advantage in both, planetary and stellar resolution, when at the seeing error level of D/r0~2. The advantage is small enough that could not materialize if the assumptions are somewhat biased toward smaller aperture.

     However, considering that most other factors - inherent optical quality, thermal equilibrium, absence of central obstruction, better collimation - generally favor smaller aperture, it makes likely the scenario suggested by the size of seeing-affected central maxima, i.e. that an aperture at D/r0 seeing level would outperform both, significantly smaller and significantly larger apertures (plots for twice smaller aperture would fit between D/r0=0 and D/r0=2, thus it is obvious that it would be outperformed by both larger apertures).

     Main difficulty in accessing more accurately effect of seeing on visual performance in apertures of different sizes is caused by the gradual, unspecified transition from seeing error effectively including only the roughness component (short-exposure error level) at small aperture sizes (i.e. at low seeing error levels, approximately D/r0<2) to also fully including the tilt error component at large seeing error level (approximately D/r0>5, long exposure error level). The dynamics of this transition can be only hinted at from the seeing MTF for both error levels over a wide range of seeing-induced error (FIG. 81).

The very nature of seeing - its constant fluctuation around the average value and, in an extended time frame, fluctuation of the average itself, benefit larger aperture more when swinging above the average. For given seeing level, D/r0 value most of the time remains within �50%, and ~25% deviations can represent appreciable portion of the time. With the seeing average following similar pattern - it can be more, or less at any given night, but this should be reasonable assumption, within the range of a rough average - r0 could reach more than two times larger size, and could be nearly twice larger over relatively significant portion of the time (on the other hand, it would be also more than twice smaller, and nearly twice smaller over nearly as much of the time).

In this particular case, with the larger-to-smaller aperture ratio 2.5, a nearly doubled r0 would put the larger aperture close to D/r0~2.5 (now closer to the short-exposure level), and the smaller one close to D/r0~1, nearly coinciding with the short-exposure level. MTF plots above indicate that it would give to the smaller aperture little in improved stellar resolution, since it already was close to 100%, while its planetary contrast would vary from that of about 1/3 smaller aperture for large details to that of nearly 90% smaller perfect aperture for planetary details near resolution threshold. The larger aperture, on the other hand, would regain most of its stellar resolution (near-equal doubles), with its planetary detail contrast at the level of about 2 times smaller perfect aperture, with its planetary detail threshold also comparable to that in a two times smaller perfect aperture. Hence, it would easily outresolve the smaller aperture on the near-equal brightness stars, while also regaining an edge in planetary contrast and resolution (effective relative aperture ~1.25D vs. 0.8D and 0.9D for planetary contrast and resolution, respectively, in the smaller aperture).

CONCLUSION

What all this implies is that it is possible for a significantly smaller aperture to outperform the larger one, but it requires them to be, and remain at a specific seeing error level, generally involving compromised seeing conditions. It is only possible when D/r0 in the larger aperture is ~4, or larger, in which case a smaller aperture with D/r0~2 will have - all else equal - better resolution and contrast transfer. In other words, smaller aperture could perform better while the seeing error is large enough, but if it lessens sufficiently due to seeing fluctuations, larger aperture would rebound and perform better. Typically, seeing fluctuations are wide enough for that to happen.

This is, of course, very simplified concept, omitting the role of other induced errors (thermals, collimation), inherent optics errors, as well as observer's individual limitations and experience, but it is necessary to assess the effect of seeing alone. Other factors can also come into play, such as luminosity of the telescopic image while observing low-contrast details. Apertures smaller than about 6 inches in diameter will provide less than optimum image brightness at high magnifications, which negatively affects eye's low-contrast detail resolution.

Following page addresses other important aspects of the seeing error: specifics of the seeing PSF, Strehl, and OTF.